Central Limit Theorem and Probability Calculations: Statistical Analysis

In this homework, we explore statistical analysis and probability through a series of questions. Each question is designed to apply statistical concepts and the Central Limit Theorem to solve real-world problems. We provide clear and structured solutions, along with a brief problem description for context, to help you understand and apply these statistical principles effectively. Let's dive into the world of data and probabilities!

Problem Description:

You are given a set of questions related to statistical analysis assignment and probability. Each question requires you to apply the Central Limit Theorem and various statistical methods to find probabilities and percentiles. Below are the solutions to each question.

Solution

Question 1

μ=30

σ=20

n=100

From the Central Limit Theorem, if a sample size is sufficiently large, n≥30, then the sample mean is approximately normally distributed with mean, μ_x ̿ =μ and standard deviation,

σ_x ̿ =σ/√n

Therefore, the sampling distribution of x ̅, has mean μ_x ̿ =30 and standard deviation,

σ_x ̿ =20/√100=2

Question 2

n=150

μ=240

σ=28

P(x ̿>4)=P(z>(239-240)/(28/√150))

=P(z>(-1)/2.28619)

=P(z>-0.4374)

=1-P(z<-0.4374)

=1-0.3309

=0.6691

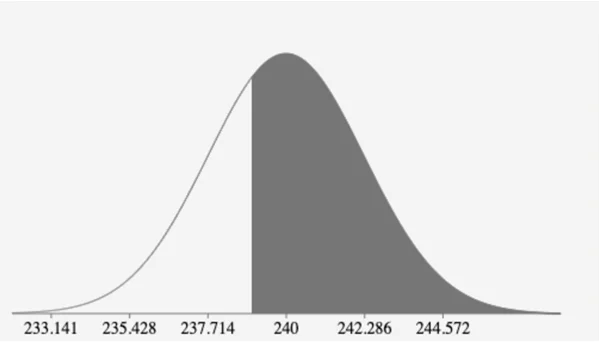
Question 2b

38th Percentile of the population

38/100=ϕ((x ̿-240)/(28/√150))

⟹ϕ^(-1) (0.38)=(x-240)/2.28619

⟹-0.305×2.28619=x-240

∴-0.6984=x-240

x=240-0.6984

=239.30

Question 2c

P(239

=P(-0.4374

=P(z<0.4374)-P(z<-0.4374)

=0.6691-0.3309

=0.3382

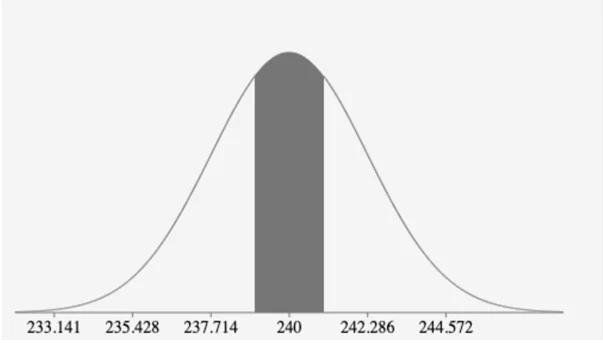
Question 2d

P(x ̿>242)=P(z>(242-240)/(28/√150))

=P(z>2/2.28619)

=P(z>0.8748)

=1-P(z<0.8748)

=0.1908

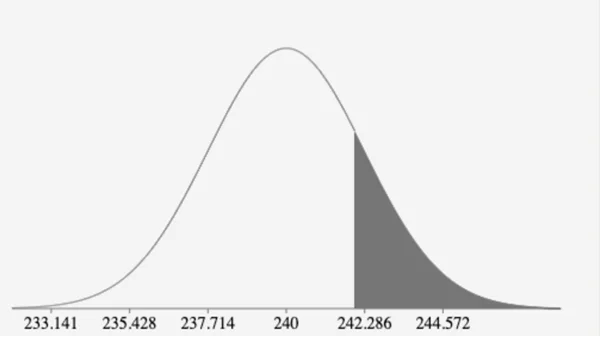
It is not unusual for the sample mean to be more than 242 since the calculated probability = 0.1908 is within the bounds of probability value; 0≤P≤1.

Question 2e

P(238

=P(-0.8748

=P(z<0)-P(z<-0.8748)

=0.5-0.1908

=0.3092

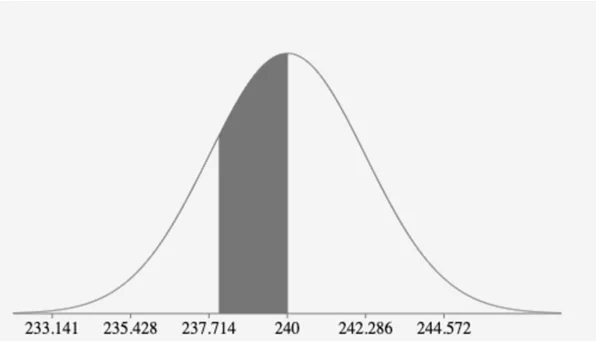
Question 3a

μ=2.25

σ=1.3

n=90

P(x ̿>2)=P(z>(2-2.25)/(1.3/√90))

=P(z>-1.8244)

=1-P(z<-1.8244)

=1-0.034

=0.966

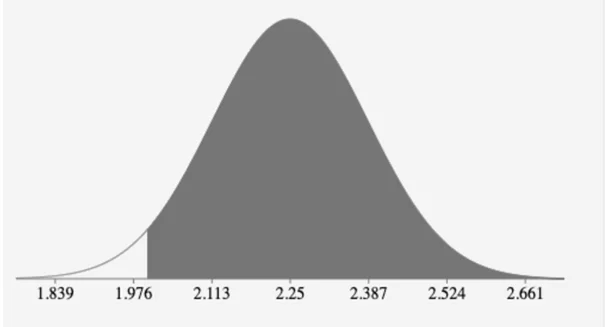
Question 3b

P(2.3

=P(0.3649

=P(z<6.9327)-P(z<0.3649)

=0.3576

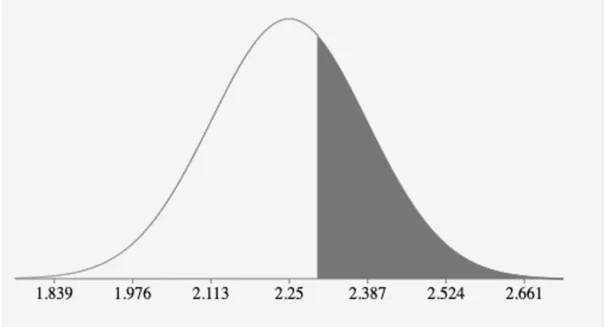
Question 3c

20th Percentile of the population

20/100=ϕ((x ̿-2.25)/(1.3/√90))

⟹ϕ^(-1) (0.2)=(x-2.25)/0.1370

⟹-0.8416×0.1370=x-2.25

∴x=2.25-0.08870

=2.16

Question 3d

P(x ̿<2)=P(z<(2-2.25)/(1.3/√90))

=P(z<-1.8244)

=0.034

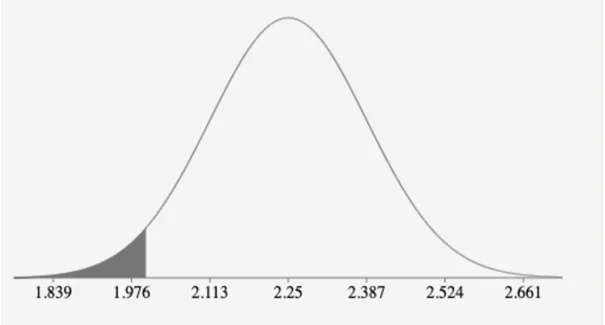
It would not be unusual for the sample mean to be less than 2 since the calculated probability =0.034 is within the bounds of probability value; 0≤P≤1.

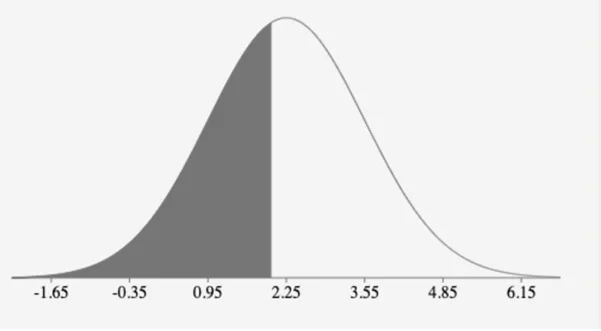
Question 3e

P(x<2)=P(z<(2-2.25)/1.3)

=P(z<-0.1923)

=P(-0.1923)

=0.4238